The Sampling Distribution of a statistic is the set of values that we would obtain if we drew an infinite number of random samples from a given population and calculated the statistic on each sample. In doing so, all samples must be of the same size (n). While it is not possible for anyone to actually draw an infinite number of samples, the concept of a sampling distribution can be understood by taking the time to carefully consider the following theoretical exercise.

Imagine that our population consists of only three numbers: the number 2, the number 3 and the number 4. Our plan is to draw an infinite number of random samples of size n = 2 and form a sampling distribution of the sample means.

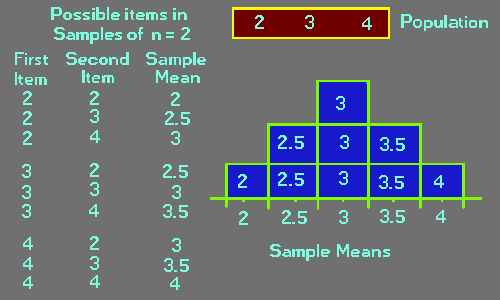
The accompanying illustration shows this population and its first two columns show each of the possible random samples (of size n = 2), that might be drawn from this population.

If the first item is a 2, the second item can be either a 2 again, or it can be a 3 or a 4. Remember! We are drawing a random sample and our population is small, hence we are sampling with replacement.

If our first item happened to be a 3, the second item might be a 2, a 3 again, or a 4 and if our first item happened to be a 4 the second item could be either a 2 or a 3 or a 4 again.

As seen here, there are only 9 possible combinations of two numbers in a given sample and each of the combinations is equally likely. The same is not true, however, for the means of the samples.

The third column in the illustration shows the means of each of the possible samples and the histogram shows the relative frequency of each of these means. In doing so the histogram provides a detailed representation of the sampling distribution of means (of size n = 2) that would be obtained if we were, in fact, able to draw an infinite number of random samples from the indicated population and graphically represent their frequency distribution in a histogram.